Question: In an orthogonal cutting, the depth of cut is halved and the feed rate is made double. If the chip thickness ratio is unaffected with the changed cutting conditions, what will be the change in actual chip thickness? [IAS 1995]

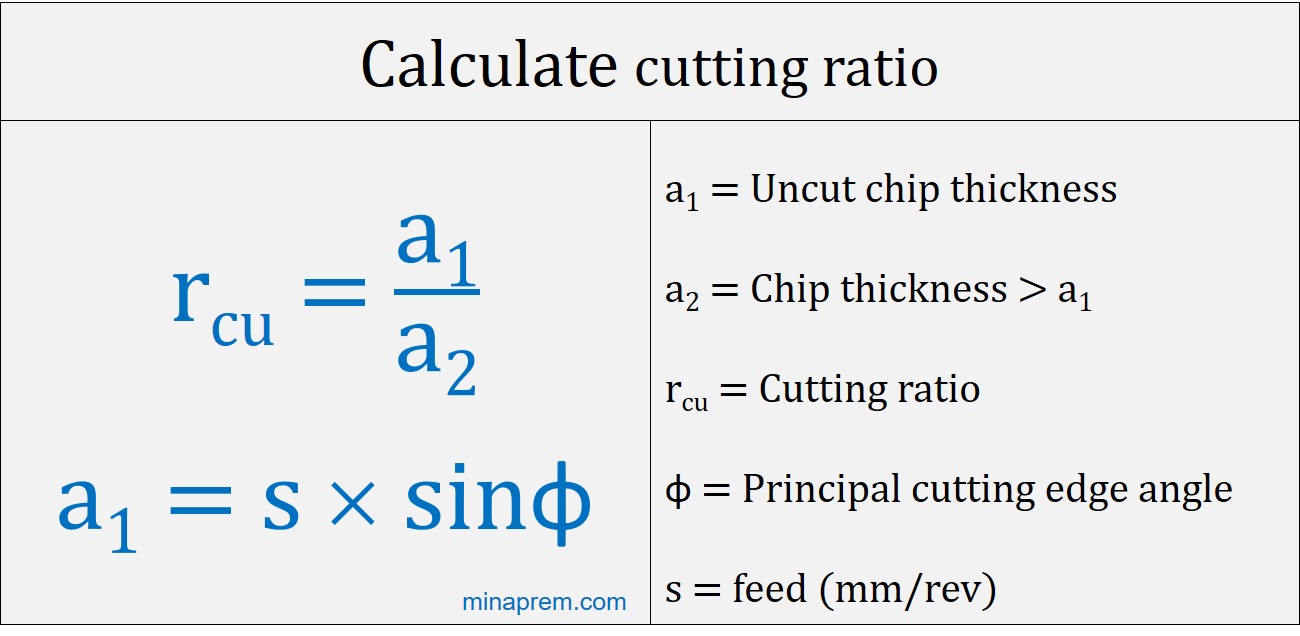
Solution: Chip thickness ratio, also called cutting ratio (r_cu), is one important parameter for machining analysis as it indicates the deformation (elongation) of chip in its thickness. Machining is basically one gradual shearing operation and thus thickness of the chip after machining becomes comparatively larger than the corresponding thickness of uncut chip. The ratio between uncut chip thickness (a₁) to the chip thickness (a₂) is called chip thickness ratio or cutting ratio (r_cu). Since chip thickness (a₂) is larger than uncut chip thickness (a₁), so chip thickness ratio is less than 1, especially for conventional macro-scale machining. Mathematically it can be expressed as given below.

\[{r_{cu}} = \frac{{{a_1}}}{{{a_2}}} < 1\]

In orthogonal turning with a single point turning tool, the uncut chip thickness (a₁) is independent of depth of cut; rather it depends on the feed and principal cutting edge angle (φ) of the tool. The width of chip is, however, a factor of depth of cut. But the chip width does not influence the chip thickness ratio; in fact, the chip width remains more or less unchanged during machining. This a₁ can be expressed in terms of feed (s) and principal cutting edge angle (φ) as given below.

\[{a_1} = s.\sin \phi \]

Determine the change in actual chip thickness

To solve the question, let us consider two cases—initial case (denoted by suffix i) and final case (denoted by suffix f). In the final case the depth of cut is halved and the feed rate is doubled but the chip thickness ratio remains unaffected. Since the tool is not changed, so the principal cutting edge angle (φ) of the cutting tool must remain unchanged. Therefore, the following can be written between the initial and final cases.

t_f = 0.5×t_i [halved depth of cut]

s_f = 2×s_i [doubled feed rate]

(r_cu)_f = (r_cu)_i [unchanged chip thickness ratio]

φ_f = φ_i [same principal cutting edge angle]

Now let us calculate the changes in actual chip thickness (a₂) based on the above conditions. This can be solved in the following way:

\[{\left( {{r_{cu}}} \right)_f} = {\left( {{r_{cu}}} \right)_i}\]

\[\frac{{{{\left( {{a_1}} \right)}_f}}}{{{{\left( {{a_2}} \right)}_f}}} = \frac{{{{\left( {{a_1}} \right)}_i}}}{{{{\left( {{a_2}} \right)}_i}}}\]

\[\frac{{{s_f}.\sin {\phi _f}}}{{{{\left( {{a_2}} \right)}_f}}} = \frac{{{s_i}.\sin {\phi _i}}}{{{{\left( {{a_2}} \right)}_i}}}\]

\[\frac{{{s_f}}}{{{{\left( {{a_2}} \right)}_f}}} = \frac{{{s_i}}}{{{{\left( {{a_2}} \right)}_i}}}\]

\[\frac{{2{s_i}}}{{{{\left( {{a_2}} \right)}_f}}} = \frac{{{s_i}}}{{{{\left( {{a_2}} \right)}_i}}}\]

\[\frac{2}{{{{\left( {{a_2}} \right)}_f}}} = \frac{1}{{{{\left( {{a_2}} \right)}_i}}}\]

\[{\left( {{a_2}} \right)_f} = 2{\left( {{a_2}} \right)_i}\]

Therefore, the actual chip thickness will be doubled for the given condition.