Question: In an orthogonal cutting operation, the tool has a rake angle of 10°. The chip thickness before the cut is 0.5mm and the cut yields a deformed chip thickness of 1.125 mm. Calculate (i) shear plane angle, and (ii) shear strain for the operation. [IAS 2015]

Solution: During machining, the excess material is gradually removed in the form of chip by shearing only. Although shearing takes place over a thick 3-D region, normally it is assumed to occur in a 2-D plane for the sake of simplicity in calculation. This plane along which shearing takes place is called shear plane. The shear plane angle is the angle of inclination of the assumed shear plane from the cutting velocity vector, as measured on orthogonal plane. It is denoted by β_O. It can be expressed in terms of Chip Reduction Coefficient (ζ) and orthogonal rake angle (γ_O), as given below.

\[\tan {\beta _O} = \frac{{\cos {\gamma _O}}}{{\zeta  – \sin {\gamma _O}}}\]

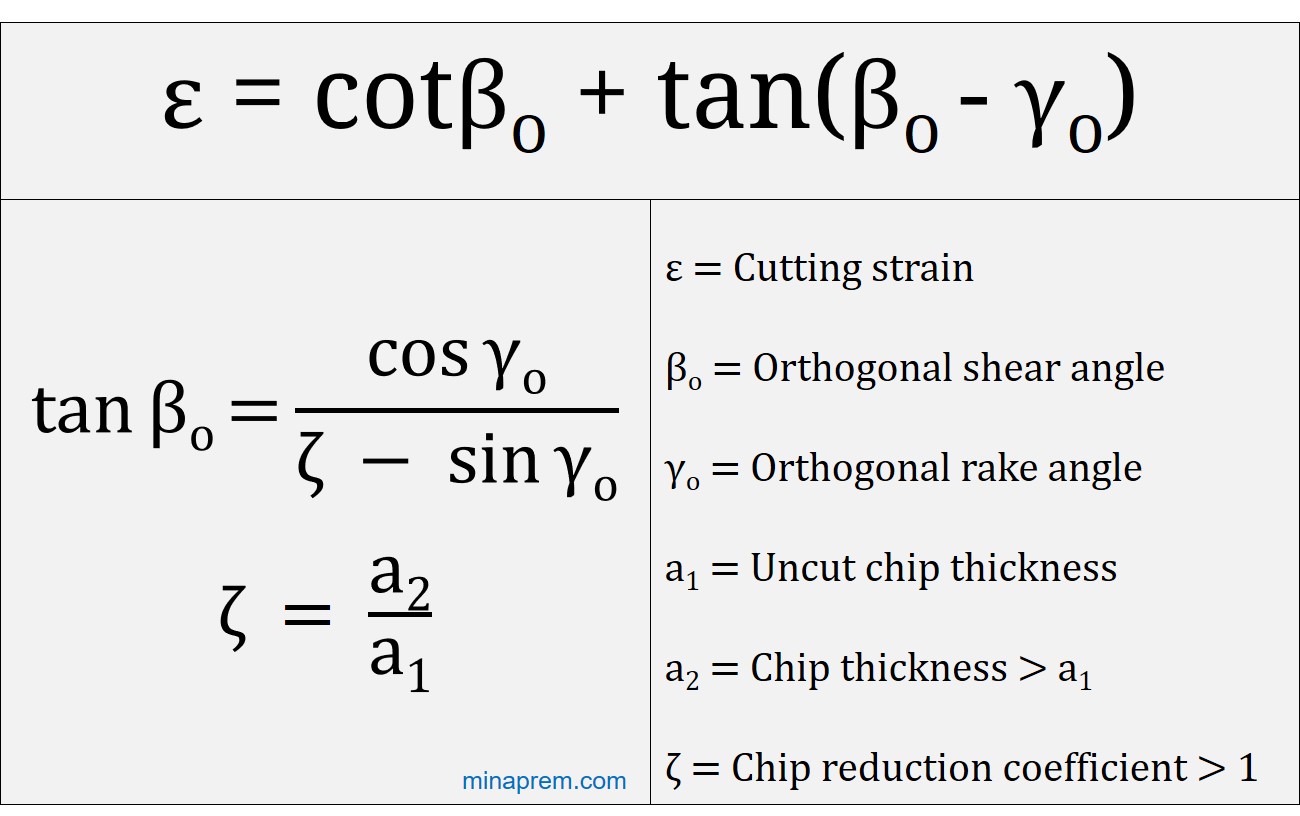
The Chip Reduction Coefficient (ζ) is the ratio between the chip thickness (a₂) to the uncut chip thickness (a₁). So it is one unitless quantity. The chip thickness is usually larger than the corresponding uncut chip thickness (i.e. a₂ > a₁) because of the positive shear strain that exists in machining. Thus Chip Reduction Coefficient is always greater than one, particularly in macro-scale machining. Mathematically,

\[\zeta  = \frac{{{a_2}}}{{{a_1}}} > 1\]

The cutting strain (ε) is the measure of average strain that induces due to shearing during machining. This strain can be expressed in terms of orthogonal rake angle (γ_O) of the cutting tool and shear angle (β_O), as given below.

\[\varepsilon  = \cot {\beta _o} + \tan ({\beta _o} – {\gamma _o})\]

Step-1: Calculate shear plane angle (β_O)

Given, orthogonal rake angle (γ_O) = +10°

Chip thickness before cut (a₁) = 0.5mm

Chip thickness (a₂) = 1.125mm

∴ Chip Reduction Coefficient (ζ) = (a₂/a₁) = (1.125/0.5) = 2.25

Substituting these values, the shear angle can be found as shown below.

\[\tan {\beta _O} = \frac{{\cos {\gamma _O}}}{{\zeta  – \sin {\gamma _O}}}\]

\[\tan {\beta _O} = \frac{{\cos 10}}{{2.25 – \sin 10}} = 0.474\]

\[{\beta _O} = {\tan ^{ – 1}}\left( {0.474} \right) = 25.37\]

Therefore, shear plane angle for the given case is 25.37°.

Step-2: Calculate shear strain in cutting (ε)

It can be easily calculated from the basic formula of cutting strain, as shown below.

\[\varepsilon  = \cot {\beta _o} + \tan ({\beta _o} – {\gamma _o})\]

\[\varepsilon  = \cot 25.37 + \tan (25.37 – 10)\]

\[\varepsilon  = 2.109 + 0.275 = 2.384\]

Hence, cutting strain for the given case is 2.384. It is unitless quantity as strain is the ratio between two lengths.