Question: A single point cutting tool with 12° orthogonal rake angle is used for orthogonal machining of a ductile material. Calculate the shear plane angle for the theoretically minimum possible shear strain. [GATE 1990]

---

Solution: During machining, a layer of uncut workpiece material gets converted into chips by shearing. Chip thickness also increases substantially compared to the same before cut. The strain that is induced because of increment in dimension due to shearing of one layer over another layer is called cutting strain or shear strain in machining. Shear strain (ε) in orthogonal turning can be expressed in terms of orthogonal shear plane angle (β_O) and orthogonal rake angle (γ_O), as given below.

\[\varepsilon  = \cot {\beta _o} + \tan ({\beta _o} – {\gamma _o})\]

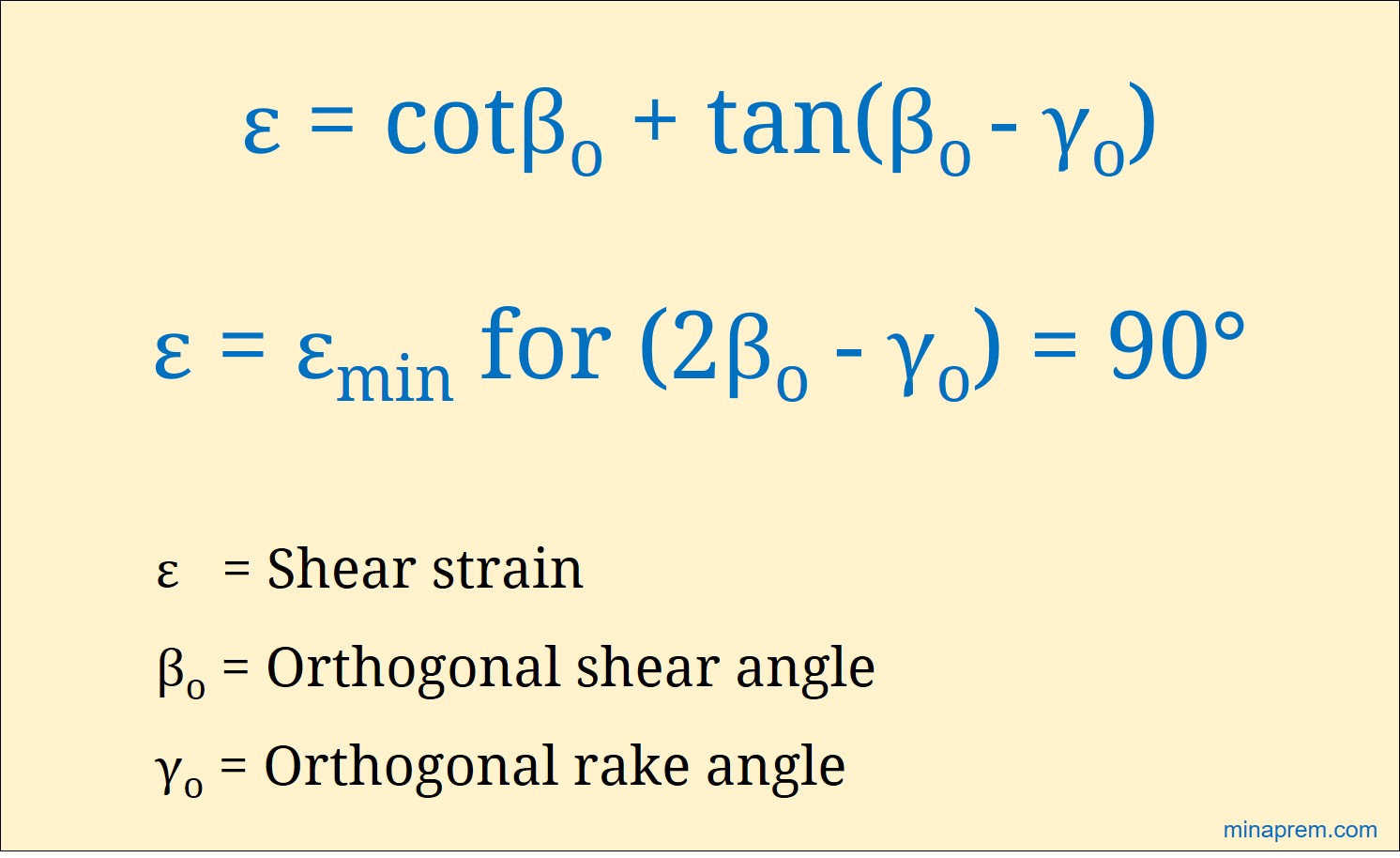
As per the given question, the objective is to get the shear angle for theoretically minimum possible shear strain when the tool has positive rake angle. To get minimum value, the shear strain is required to differentiate with respect to β_O. The first order differentiation and second order differentiation are given below.

First order differentiation

\[\frac{{d\varepsilon }}{{d{\beta _o}}} = \frac{d}{{dx}}\left[ {\cot {\beta _o} + \tan ({\beta _o} – {\gamma _o})} \right]\]

\[\frac{{d\varepsilon }}{{d{\beta _o}}} =  – \frac{1}{{{{\sin }^2}{\beta _o}}} + \frac{1}{{{{\cos }^2}({\beta _o} – {\gamma _o})}}\]

Second order differentiation

\[\frac{{{d^2}\varepsilon }}{{d{\beta _o}^2}} = \frac{{2\sin {\beta _o}\cos {\beta _o}}}{{{{\sin }^4}{\beta _o}}} + \frac{{2\cos ({\beta _o} – {\gamma _o})\sin ({\beta _o} – {\gamma _o})}}{{{{\cos }^4}({\beta _o} – {\gamma _o})}}\]

\[\frac{{{d^2}\varepsilon }}{{d{\beta _o}^2}} = \frac{{\sin 2{\beta _o}}}{{{{\sin }^4}{\beta _o}}} + \frac{{\sin (2{\beta _o} – 2{\gamma _o})}}{{{{\cos }^4}({\beta _o} – {\gamma _o})}}\]

Calculate shear angle for minimum shear strain

In order to get minimum shear strain (ε), the first order differentiation is made equal to zero. Thus, (dε/dβ_o) = 0 leads to:

\( – \frac{1}{{{{\sin }^2}{\beta _o}}} + \frac{1}{{{{\cos }^2}({\beta _o} – {\gamma _o})}} = 0\)

or, \(\frac{1}{{{{\sin }^2}{\beta _o}}} = \frac{1}{{{{\cos }^2}({\beta _o} – {\gamma _o})}}\)

or, \({\sin ^2}{\beta _o} = {\cos ^2}({\beta _o} – {\gamma _o})\)

or, \(\sin {\beta _o} = \cos ({\beta _o} – {\gamma _o})\) [∵ 0° < β_O <90°]

or, \(\cos (90 – {\beta _o}) = \cos ({\beta _o} – {\gamma _o})\)

or, \((90 – {\beta _o}) = ({\beta _o} – {\gamma _o})\)

or, \(2{\beta _o} = 90 + {\gamma _o}\)

or, \({\beta _o} = \left( {90 + 12} \right)/2 = {51^ \circ }\) [Given, γ_O = +12°]

Check whether minimum or maximum

For a cutting tool with orthogonal rake angle of +12°, either minimum or maximum shear strain will be obtained when shear angle is +51°. If the second order differentiation at β_O = +51° and γ_O = +12° is a positive value then shear strain will be minimum. So let us check (d²ε/dβ_o²) value for the above conditions using the second order differential equation as derived earlier in order to validate minimality of strain.

\[\frac{{{d^2}\varepsilon }}{{d{\beta _o}^2}} = \frac{{\sin 102}}{{{{\sin }^4}51}} + \frac{{\sin (102 – 24)}}{{{{\cos }^4}(51 – 12)}}\]

\[\frac{{{d^2}\varepsilon }}{{d{\beta _o}^2}} = 5.36 > 0\]

Since the minimality condition is satisfied, so the minimum shear strain in orthogonal turning with a cutter having +12° orthogonal rake angle will be obtained at shear angle of β_O = +51° (Answer).

Value of minimum shear strain

This is an additional part, as the question did not asked for it. In case the value of minimum shear strain (ε_min) is also required to calculate then it can be easily found out from the general formula for shear strain (ε) as mentioned at the beginning. Here, γ_O = +12° and β_O = +51°.

\[{\varepsilon _{\min }} = \cot 51 + \tan (51 – 12)\]

\[{\varepsilon _{\min }} = 0.81 + 0.81 = 1.62\]

Therefore, for the given case, the minimum shear strain is 1.62.