Question: In a pure orthogonal turning process, the chip thickness is 0.32mm, feed is 0.2 mm/rev. What is the cutting ratio? [ESE 2014]

Solution: The ratio between the uncut chip thickness (a₁) to the chip thickness (a₂) is termed as cutting ratio (r_cu). Owing to lamellar shearing and positive strain, the chip thickness increases as compared to the same for uncut chip. Thus cutting ratio is smaller tan 1, especially for conventional macro scale cutting. Mathematically, cutting ratio (r_cu) can be expressed as follows:

\[{r_{cu}} = \frac{{{a_1}}}{{{a_2}}} < 1\]

However, in the question, the uncut chip thickness (a₁) is not specifically mentioned; instead the feed is given. The machining operation is pure orthogonal turning process. Thus principal cutting edge angle (φ) of the tool is 90°. In case of turning, the uncut chip thickness (a₁) can be expressed in terms of feed (s) and principal cutting edge angle (φ), as given below. Note that feed has to be specified in mm/rev unit; but not in mm/min unit that is also used for feed rate in turning.

\[{a_1} = s.\sin \phi \]

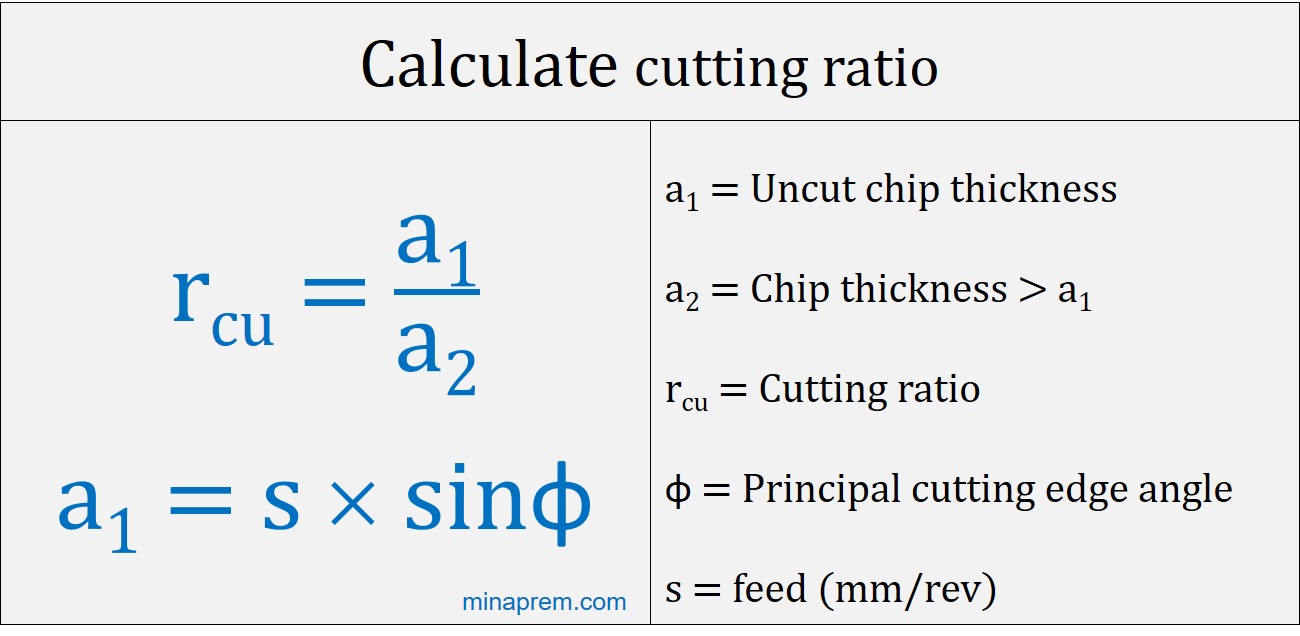
Determine cutting ratio for the given case

Chip thickness (a₂) = 0.32mm

Principal cutting edge angle (φ) = 90° [∵ Pure orthogonal turning]

Feed (s) = 0.2mm/rev

∴ Uncut chip thickness (a₁) = s × sinφ = 0.2 × sin90 = 0.2mm

Hence, the cutting ratio be:

\[{r_{cu}} = \frac{{{a_1}}}{{{a_2}}} = \frac{{0.2}}{{0.32}} = 0.625\]

Therefore, cutting ratio for the given case is 0.625. It is one unitless quantity as it is the ratio of two lengths.